Geometry: from Greek, gē ‘earth’ + metria 'measure'

Geometry literally translates as “to measure the earth,” and it gives us the tools to do just that, or, more precisely, it gives us consistent mathematical models with which to measure complex phenomena. How can teachers take geometry lessons beyond the plane and solid figures in the classroom to recognize and understand the underlying mathematical mechanisms of natural and human-created forms? This article examines some of the ideas and observations often referred to as sacred geometry and discusses ways in which elementary students can explore these topics using principles of mathematics. Beyond these examples, we also discuss the following:

• What is sacred geometry?
• How does sacred geometry apply to Psychogeometry?
• Is sacred geometry compatible with the curriculum in Montessori secular classrooms?

The Levels of Montessori Geometry

In Part One of this series, we saw that students in the Montessori classroom learn geometry concepts through their exploration of plane figures and solids. To review, these explorations are largely sensorial at the Early Childhood (EC) level, while students in the Elementary classroom begin to abstractly understand the relationships inherent within and between planes and solids. To illustrate, while the EC child learns sensorially that two triangles placed together form some sort of quadrilateral, the Elementary child can deduce that since the sum of the angles of any triangle is 180º, the sum of the angles of any quadrilateral, formed by two triangles (180º x 2), must be 360º. This reflects the three levels of learning geometry that Montessori discusses in Psychogeometry: sensorially exploring, reasoning through a concept, and finally deriving a formula and measuring (Lockhart, 2019).

These are important experiences for children in the Elementary program; however, some students might not move beyond the Seven Triangles of Reality work if geometry is relegated to the back burner of the curriculum. Teachers who may have found geometry a difficult or uninspiring subject in high school may intentionally or otherwise avoid it, with the regrettable consequence that some children miss out on the second and third levels of learning geometry—those which promote “higher-order thinking, observation skills, and mastery of concepts that both meet current standards and provide knowledge that many outside of Montessori will not encounter until middle or high school” (Lockhart 2019, iii). Perhaps even more unfortunate is that they miss out on early opportunities to apply geometric principles to understanding the world around them.

Beyond Planes and Solids

The Montessori geometry exercises are designed for the child to discover relationships between figures, but there is great value for both teachers and students in moving beyond the planes and solids to discovering and appreciating the geometric principles underlying natural and human created forms. Geometry gives us another lens through which to view the world, one that helps us to recognize the mathematical basis of patterns and forms, and also to understand the complex relationships among and between these patterns and forms. The value of geometry to this end is expressed by Harvard mathematician Shing-Tung Yau:

...geometry is one of the main avenues available to us for probing the universe. Physics and cosmology have been, almost by definition, absolutely crucial for making sense of the universe. Geometry's role in this may be less obvious, but is equally vital. I would go so far as to say that geometry not only deserves a place at the table alongside physics and cosmology, but in many ways it is the table. (2010, 18).

Sacred Geometry

The growth spiral of a nautilus shell, the symmetry of a snowflake, the tessellations of a honeycomb—these and other naturally occurring forms and patterns have been interpreted by some—historically and presently—as sacred geometry. Beautiful and compelling, these forms reflect specific geometric proportions to which philosophers and scientists of the classical age (and others today) ascribed sacred symbolism and attributed to a divine geometer. Despite Montessori's Catholic background, she did not write about sacred geometry as such—which is likely “a modern moniker” (Lockhart, 2021). She did, however, give geometry an important role in Cosmic Education, in her definition of psychogeometry as the “measurement of the Earth together with the consciousness of the reciprocal relationship between Man and the objects of the environment, and between the objects themselves” (1934, 2011).

According to Bendetto Scoppola, math professor at the University of Rome and editor of Psychogeometry, Montessori seems to want to present Euclidean geometry to children with a Platonic attitude. As evidence of the presence of Euclid in the curriculum, Scoppola (2013) points to Montessori's translation of Euclid's 47th proposition into a classic geometry material. The proposition states: “In right-angled triangles the square on the side opposite the right angle equals the sum of the squares on the sides containing the right angle” (also known as the Pythagorean theorem). The material brilliantly proves the theorem:

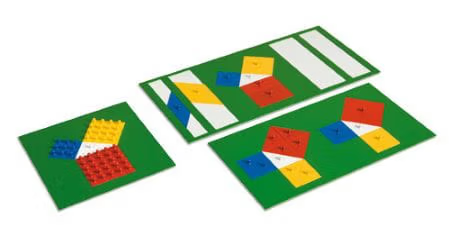
Contrast Euclidean geometry with Mathematical Platonism: “... the metaphysical view that there are abstract mathematical objects whose existence is independent of us and our language, thought, and practices … Mathematical truths are therefore discovered, not invented” (Stanford Encyclopedia of Philosophy 2018). In other words, the truth of the famous theorem—its inherent properties—existed before and is independent of its description and proof by the likes of Pythagoras and Euclid.

The Platonic solids provide another example. The tetrahedron, hexahedron, octahedron, dodecahedron, and icosahedron, each with congruent regular polygonal faces with the same number of faces meeting at each vertex, are the only possible convex solids. Plato found the idea of exactly five possible convex solids very compelling and concluded they must represent the elements of fire, earth, air, and water, plus spirit. This idea certainly has applications to Cosmic Education and provides a link (in Platonic thinking) between geometry and all things in the universe. On the other hand, the solids also lend themselves to such exploratory and mathematical work as devising nets for their construction and extrapolating the formula for the relationship of edges, faces and vertices (Euler's formula).

Montessori clearly wants children “to discover things with a material, Platonic attitude, and then [move on to] rigorous constructions” (Scoppola, 2013). By designing the curriculum around Euclid's Elements, however, she also wants to prepare the mind of the child for rational thought. Scoppola suggests that rationality is an important element of Montessori's peace education, in that irrationality can lead to the type of fundamentalism that runs counter to a peaceful world—an intriguing idea that exceeds the limitations of this article.

Sacred Geometry in Montessori Classrooms

We can teach about specific mathematical topics, sometimes described as “sacred geometry,” in Montessori classrooms (especially Upper Elementary), not to impart a religious or otherwise ideological lesson, but to help children understand a very complex world and the complex relationships within that world. As Betsy Lockhart tells her students, “Geometry is the language of the universe, and math is our attempt to describe or reproduce that geometry” (2021). By teaching children the mechanisms underlying the spiral formation of a pinecone or hurricane, the fractal branching of trees and river systems, or the arrangement of corn kernels on a cob and spots on a giraffe, we give them the tools to make comparisons, find commonalities, and discover relationships.

Some mathematical sequences have been found to model natural patterns; e.g., the Fibonacci sequence. To illustrate, let's examine the relationship between the Fibonacci sequence, spirals, and the golden ratio—an inspiring and logical mathematical trio. The Fibonacci sequence is created by starting with 0 and 1, then adding the two preceding numbers to get the next one in the series: 0+1=1; 1+1=2; 1+2=3; 2+3=5; 3+5=8, etc. The sequence (0, 1, 1, 2, 3, 5, 8, 13, 21, 34 … nx) is a mathematical model for the growth factor of spirals and is also reflected in the golden ratio.

The golden ratio refers to a unique relationship between two numbers, symbolized using φ (phi), the 21st letter of the Greek alphabet (see image below). Frequently expressed in rectangles, the golden ratio's proportion is considered so aesthetically pleasing that it is frequently used in art and architecture, intentionally or not, in the likes of the Parthenon, Michaelangelo's Sistine Chapel, and in da Vinci's most renowned works. It is noteworthy, however, that this idea is quite contentious, with some mathematicians pointing out that superimposing the proportions of the golden ratio over a piece of art or photo of a structure may be more a simple projection than a strict application of mathematics, constituting what has been referred to as “unanchored geometry” (Skinner, 2006).

Spirals can be found in plants (spiral aloe leaves, sunflower seed heads); animals (mollusk shells, the horns of bighorn sheep); microorganisms (spiraling bacteria), the atmosphere (hurricanes), and space (galaxies). Fascinating in their own right, their mathematical representation by the Fibonacci sequence adds to their elegance. The series identifies, for example, the number of leaves or seeds at the successive opposing twists of each spiral. When studied from a physics or mathematical perspective, children might explore the unique conditions under which different spirals originate; each has its own genesis—the leaf arrangement in protein folding, the galaxy in a vacuum, and a hurricane in the atmosphere.

The following image presents a visual representation of a golden rectangle and its relationship to the Fibonacci sequence and spirals:

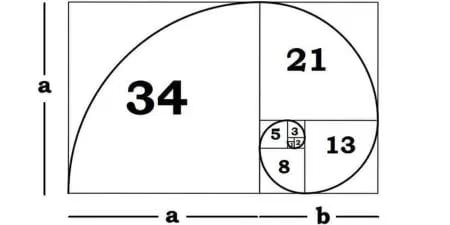
Exploring these and other phenomena takes the study of geometry into another realm—an integration of micro- and macro-phenomena—and helps children learn to recognize its expression in the world. Learning and applying the mathematics underlying these forms helps them see the commonality within the forms' inherent structures without projecting sacredness, which may obfuscate more straightforward geometry lessons. This does not mean, however, that these forms cannot also be appreciated from a purely aesthetic perspective and for their relatedness to other natural phenomena.

The classroom applications of this work range from a concrete exploration of the forms through photos and actual plant and animal specimens for younger children, to fraction and decimal work for older children. Upper Elementary students can, for example, construct and deconstruct a golden rectangle, make calculations with the Fibonacci sequence, and identify examples of both in nature and architecture (Lockhart, 2021). Additionally, many of these activities align with the Next Generation Science Standards.

Children will also enjoy exploring other numbers and patterns in nature, such as symmetry, fractal branching, tessellations, cracks, flows and meanders, bubbles and foam, waves and dunes, and spots and stripes. Clearly, these topics can and should be connected to other areas in the classroom—art, botany, chemistry, physics, zoology. Like any area in the curriculum, children will find more meaning and relevance in their geometry and math studies when they make connections and find relationships.

When we move beyond the planes and solids, geometry reveals the wonders of the world and gives us the tools with which to construct our knowledge of the world. Whether sacred or mathematical, or both, the expression of geometry is all around us, in both natural and human created forms. “Patterns surround us in nature. They'll tell you how things work on our planet, if you know where to look to find them” (Museum of Science+Industry Chicago 2021).

References

Lockhart, Betsy. (owner and consultant, Lockhart Learning), in discussion with the author. September 2021.

Montessori, M. (1934/2011). Psychogeometry (Montessori Series Volume 16). Netherlands: Montessori-Pierson Publishing Company.

Shing-Tung Yau and Nadis, S. (2010). The shape of inner space. New York: Basic Books.

Skinner, S. (2006). Sacred geometry: deciphering the code. New York: Sterling Publishing Co., Inc.

About the Author

Cynthia Brunold-Conesa, MEd, is an educator of adult learners at two AMS teacher education programs. She has 23 years experience as a lead guide at the Elementary and middle school levels. Cynthia also publishes on a variety of Montessori topics. She is AMS credentialed (Elementary I – II). Contact her at cynthia.conesa@meipn.org .

Interested in writing a guest post for our blog? Let us know!

The opinions expressed in Montessori Life are those of the authors and do not necessarily represent the position of AMS.